- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2015
- Matter at extremes
- Unconventional high-Tc superconductivity in fullerides
Unconventional high-Tc superconductivity in fullerides
Molecular superconductors such as the fullerides are characterised by periodic arrangements of molecules. Here, for the A3C60 fullerides, Tc was found to have a dome-like variation with the density of fulleride molecules, controlled by pressure, and that the peak of this dome occurs precisely at the point where the molecular and extended lattice features of the electronic structure are optimally balanced.
Fulleride superconductors are the simplest members of the unconventional superconductivity family, i.e. the pairing interaction is something other than simply the conventional BCS electron-phonon interaction. This was demonstrated by showing that, in both polymorphs (A15− and face-centred-cubic−structured) of the most expanded binary fulleride Cs3C60, superconductivity emerges upon applied pressure out of an antiferromagnetic Mott-Jahn-Teller insulating state and displays an unconventional behaviour – a superconductivity dome – explicable by the prominent role of strong electron correlations [1-5]. However, although the similarities with the electronic phase diagrams of exotic superconductors such as the cuprates, the iron pnictides, and the heavy fermions are striking, there existed to date no information on the nature of the parent normal metallic state out of which both the insulating and superconducting states of fullerides emerge. The relation of this parent state to both the superconducting pairing mechanism and the proximate antiferromagnetic insulator is at the heart of any attempt to understand unconventional superconductivity and has been pursued for decades in the cuprates. The high symmetry and structural simplicity of the fullerides are advantageous in pursuing a direct connection from (molecular) building unit to extended structure properties.
 |
|
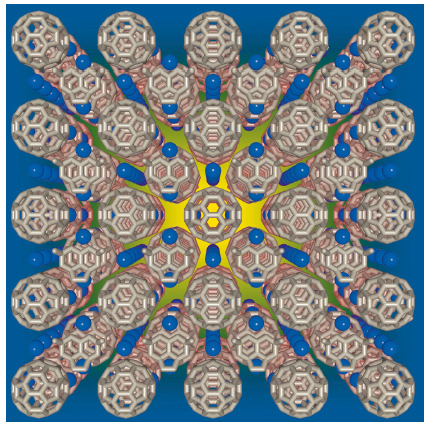
Fig. 104: Three-dimensional depiction of the crystal structure of the molecular A3C60 fulleride superconductors. The fullerene molecules pack in an isotropic cubic-close-packed array in three dimensions. Alkali metal ions (blue spheres) occupy vacant interstitial holes of octahedral and tetrahedral symmetry. |
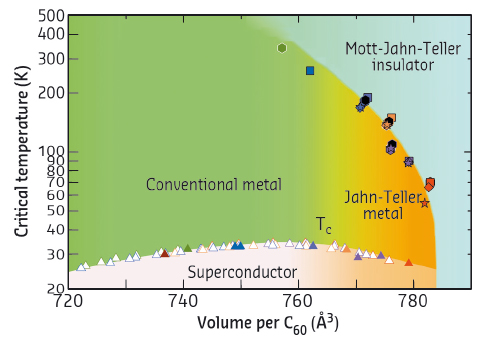
We deployed a panoply of experimental techniques to probe the evolution of the electronic and crystal structure changes associated with the Mott insulator-metal transition in fcc-structured fullerides (Figure 104). The entire bandwidth-controlled electronic phase diagram can be traversed by changing an electronic parameter − the extent of overlap between the outer wavefunctions of the fulleride anions − as monitored by the application of (physical or chemical) pressure. Synchrotron X-ray powder diffraction measurements at both ambient (beamline ID31, now ID22) and elevated pressures (beamline ID27) down to temperatures below the observed Tc show that the high-symmetry isotropic fcc structure remains robust at all relevant values of temperature and pressure and allow us to extract the dependence of the electronic properties on C60 packing density V, which in turn accurately tracks experimentally the change in bandwidth. Combining the structural results with those from spectroscopic and thermodynamic probes demonstrated for the first time the influence of the molecular origin of the electrons at the Fermi level on the collective transport and superconducting pairing properties. We found an anomalous correlated metallic phase – the Jahn-Teller metal – which dominates the phase diagram at temperatures well above Tc and is the normal state at the top of the superconductivity dome when Tc is maximum and where the superconducting pairing crosses over from conventional weak-coupling to unconventional strong coupling (Figure 105). The Jahn-Teller metal is a dynamic, microscopically heterogeneous coexistence of itinerant metallic electrons with localised electrons, which produce Jahn-Teller on-molecule distortions driven by the degeneracy of the frontier orbitals of the C60 building unit. This central electronic state is the parent of an unconventional strongly-coupled superconducting state and fades away into a conventional Fermi liquid metal and weak-coupling BCS superconductor when the molecular electronic signatures disappear with lattice contraction.
 |
|
Fig. 105: Global electronic phase diagram of fcc-structured A3C60 fullerides showing the evolution of the superconducting transition temperature, Tc (superconductivity dome) and the Mott-Jahn-Teller insulator to Jahn-Teller metal crossover temperature, T’ as a function of volume per C60. Within the metallic (superconducting) regime, gradient shading from orange to green schematically illustrates the Jahn-Teller metal to conventional metal (unconventional to weak-coupling BCS conventional superconductor) crossover. |
The optimal Tc in the fullerides – and highest for any molecular material – is found precisely at the boundary between unconventional and conventional normal and superconducting state behaviour, where the balance between molecular (Jahn-Teller distortion) and extended lattice (itinerant metallic electrons) features of the electronic structure is optimised.
Principal publication and authors
Optimized unconventional superconductivity in a molecular Jahn-Teller metal, R.H. Zadik (a), Y. Takabayashi (a), G. Klupp (a,b), R.H. Colman (a), A.Y. Ganin (c), A. Potočnik (d), P. Jeglič (d), D. Arčon (d), P. Matus (b), K. Kamarás (b), Y. Kasahara (e), Y. Iwasa (e), A.N. Fitch (f), Y. Ohishi (g), G. Garbarino (f), K. Kato (h), M.J. Rosseinsky (c) and K. Prassides (a,i,j), Science Advances 1, e150059 (2015); doi: 10.1126/sciadv.1500059.
(a) Department of Chemistry, Durham University (UK)
(b) Institute for Solid State Physics and Optics, Wigner Research Centre for Physics, Hungarian Academy of Sciences, Budapest (Hungary)
(c) Department of Chemistry, University of Liverpool (UK)
(d) Institute Jožef Stefan, Ljubljana (Slovenia)
(e) Quantum-Phase Electronics Center and Department of Applied Physics, University of Tokyo (Japan)
(f) ESRF
(g) Japan Synchrotron Radiation Research Institute, SPring-8, Hyogo (Japan)
(h) RIKEN SPring-8 Center, Hyogo (Japan)
(i) WPI-Advanced Institute for Materials Research (WPI-AIMR), Tohoku University, Sendai (Japan)
(j) Japan Science and Technology Agency (JST), ERATO Isobe Degenerate π-Integration Project, Tohoku University, Sendai (Japan)
References
[1] A.Y. Ganin et al., Nature Mater. 7, 367 (2008).
[2] Y. Takabayashi et al., Science 323, 1585 (2009).
[3] A.Y. Ganin et al., Nature 466, 221 (2010).
[4] G. Klupp et al., Nature Commun. 3, 912 (2012).
[5] A. Potočnik et al., Sci. Rep. 4, 4265 (2014).



