- Home
- Users & Science
- Find a beamline
- Structure of materials
- ID19 - Microtomography beamline
- ID19 Techniques
- Diffraction Topography
- X-ray diffraction imaging (X-ray topography)
X-ray diffraction imaging (X-ray topography)
An Overview of Techniques and Applications
Contents
-
1. Introduction
2. Basic diffraction topographic techniques-
2.1 Extended beam (wide area) methods
-
2.1.1 Integrated wave topography
-
a) White beam topography
b) Lang (projection) topography
2.1.2 Double crystal topography -
a) White beam topography
2.2 Section topography (limited beam method) -
2.1.1 Integrated wave topography
3. Basic origins of contrast
-
a) Structure factor contrast
b) Orientation contrast
c) Extinction contrast
4. X-ray topography on thin layers
5. New possibilities of modern synchrotrons
6. Literature -
2.1 Extended beam (wide area) methods
X-ray diffraction topography is an imaging technique based on Bragg diffraction (Bragg’s law l = 2 dhkl sinQ B, with l - X-ray wavelength, dhkl - lattice plane spacing, QB - Bragg angle). It provides a two dimensional intensity mapping of the beam(s) diffracted by a crystal.
It is used for the visualisation of defects (dislocations, twins, domain walls, inclusions, impurity distribution, …) present in the crystal volume. More exactly, it records the long range distortion fields and/or the strain fields associated with a macroscopic crystal deformation (e.g. bending, "heat bump"). This becomes possible, because these distortion fields may affect the diffracted intensity, so give "contrast" (non-homogeneous intensity distribution) in the image. In this way topography is a study of the fine structure of a Bragg spot which contains the information about the departures from the perfect crystal structure (which is investigated by structure determination methods and assumed as known), that is the defect structure.
The basic principle of X-ray topography is shown in Fig.1. References and further details may be found e.g. in [1-3].
| a) |
 |
|
| b) |
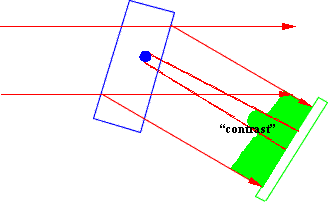 |
Fig. 1: Basic principle of X-ray diffraction topography for an extended (homogeneous) beam. Situation for a perfect a) and an imperfect crystal b).
2. Basic diffraction topographic techniques
The classification of the different topographic methods depends on three basic elements:
i) the used source geometry and characteristics, including possible optical elements (slits, other crystals, …),
ii) the sample properties and geometry, and
iii) the detector, also including possible optical elements.
Only a limited selection of these techniques is of importance from the today and practical point of view. We will show the general physical properties and ideas and concentrate on the techniques applied at synchrotrons. It should be mentioned, that all techniques may be used in the transmission (Laue) or in the reflection (Bragg) case.
2.1 Extended beam (wide area) methods
An extended beam is such that a beam diffracted by a perfect crystal plate (and a possible additional integration on the detector - compare Lang technique, compare paragraph 2.1.1.b) shows homogeneous intensity, that is, no interference fringes are visible (schematic view in fig. 2). For a general quantitative criterion we have to take in consideration the diffraction geometry, crystal and source parameters, but for a simple estimation e.g. in the symmetrical Laue case, one can use the following condition for the beam width (in the diffracting plane) b:
b >> 2 t sinQ B ,
where t is the crystal thickness.
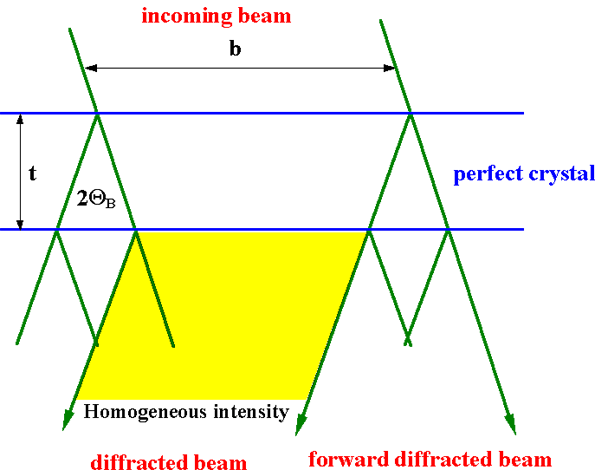
Fig. 2: Schematic "definition" of an extended beam (example for the symmetrical Laue or transmission case)
2.1.1 Integrated wave topography
Integrated wave topography means that the topograph recorded with such a technique is not created by a single plane, monochromatic wave or by a cylindrical, monochromatic wave (which are the fundamental ones from the physical and theoretical point of view), but by a superposition of such waves. That means that the incoming beam must be divergent and/or non-monochromatic. A suited reference for these beam properties are the full width at half maximum of a reflectivity curve
- in the angular space:
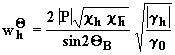
- and in the wavelength space:
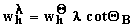
with: P - polarisation factor, 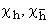 - Fourier components of the electric susceptibility, with, g0=sin(a+QB), gh sin(a-QB) - direction cosines of the forward diffracted and diffracted beam, a - the angle between crystal surface and reflecting lattice planes. The relation of ch with the structure factor Fh is ch = - [(r0l2)/(pVuc)] Fh, where r0 is the classical electron radius and Vuc the volume of the unit cell.
- Fourier components of the electric susceptibility, with, g0=sin(a+QB), gh sin(a-QB) - direction cosines of the forward diffracted and diffracted beam, a - the angle between crystal surface and reflecting lattice planes. The relation of ch with the structure factor Fh is ch = - [(r0l2)/(pVuc)] Fh, where r0 is the classical electron radius and Vuc the volume of the unit cell.
These values whQ and whl are to be compared with the angular source size d (the angular size of the source seen from one point of the crystal, that is d = s/L0, where s is the geometrical source size and L0 the source to crystal distance) and the spectral width Dl s of the incoming beam.
The conditions that such an integration for generating an integrated wave topograph may take place are:
d > w h Q and/or D l s > w h l
Table 1 provides an idea about the magnitudes of those parameters.
| energy | whQ | whl/l |
| 8 keV | 7.6 arcsec | 1.5·10-4 |
| 20 keV | 2.9 arcsec | 1.5·10-4 |
Table 1: Intrinsic full width at half maximum of the reflectivity curve in the angular, w h Q , and in the wavelength, w h l, spaces (the last normalised), as a function of energy for a thick silicon crystal and the 111-reflection in the Bragg case.
| source | source size s | source distance L0 |
angular source size d |
| laboratory | 400 mm | 0.4 m | » 1·10-3»3.5 arcmin |
| ID19 | 100 mm | 150 m | » 6.7·10-7»0.14 arcsec |
Table 2: Angular source size as a function of typical parameters for a laboratory source and for the ID19 synchrotron beamline.
| source | Dls/l |
| laboratory, (e.g. CuKa1) | 3·10-4 |
| SR, Si 111 double monochromator | 2·10-4 |
| white beam | 1···10 |
Table 3: The normalised spectral width, Dl s, for the beam from a laboratory source, a "monochromatised" synchrotron beam, and a "white" synchrotron beam.
Thus, we may conclude the following: concerning the divergence:
 laboratory sources:
laboratory sources:
d >> whQthey produce exclusively divergent waves beamline ID19 :
d << whQon most cases we have quasi plane waves
concerning monochromaticity:
 laboratory source, synchrotron source in "monochromatic" mode:
laboratory source, synchrotron source in "monochromatic" mode:
three cases are possible:
Dls < whl monochromatic waves (rare case)
Dls » whl transition case
Dls > whl non-monochromatic (more often case)
synchrotron source in white beam mode:
Dls >>> whlwhite beam, always non-monochromatic
a) White beam topography
White beam topography is a powerful and very simple technique when using synchrotron radiation. The basic arrangement for this technique is trivial. It is practically the one utilised in 1912 for the first X-ray diffraction experiment (Laue technique, used commonly e.g. for structure analysis or crystal orientation) with the "only" difference that a wide, homogeneous,
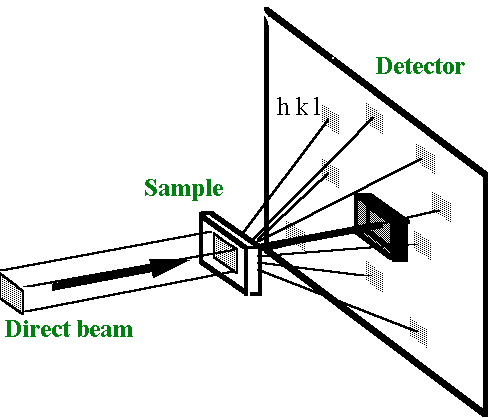
Figure 3a: White beam topographic set-up
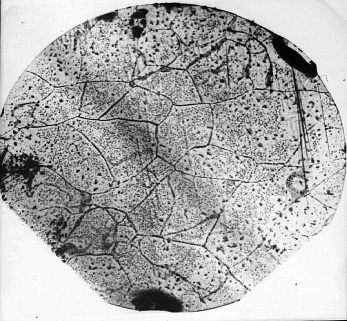
Figure 3b: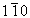 white beam topograph of a (001) Fe-3% Si crystal (l» 0.04 nm, X-ray generator with a silver target).
white beam topograph of a (001) Fe-3% Si crystal (l» 0.04 nm, X-ray generator with a silver target).
low divergent and powerful beam is applied (fig. 3a). Examples of white beam topography was used first with laboratory sources [4, 5] (Bremsspectrum), but now it is mostly used with synchrotron radiation [6]. This allows to record white beam topographs with exposure times between milliseconds and some minutes. It is clear that the condition Dls > whl is fulfilled without problems. In addition we have in general a quasi parallel beam, because d << whQ . In this way the image recorded by the detector is an integration of (quasi) plane, monochromatic waves over the wavelength. The above mentioned second condition is not compulsory (but fulfilled for modern synchrotrons with small source dimensions and large distances to the experimental stations) for the diffracted intensity distribution at the exit surface of the crystal, because this results in a second, redundant integration of the same character. However, a small value d leads to a very good geometrical resolution r (fig. 4)
r = s D / L0 = d D

Figure 4: Geometrical resolution
on the detector and allows to use large sample to detector distances. This is important for several reasons. It allows:
1) background reduction (sample fluorescence, necessary e.g. for GaAs samples) ,
2) distinguishing between orientation and extinction contrast (see below) by recording,
topographs very close (extinction contrast is pronounced) and very far from the sample
(orientation contrast is pronounced).
In addition the small angular source size leads to very good beam coherence properties (see related chapter).
An example of a white beam topograph of a part of a low dose SIMOX wafer (special technology to produce a thin silicon layer on a silicon substrate with a thin Silicon oxide layer in between them, produced by implantation of oxygen) is shown in figure 5 (W. Ludwig, J. Härtwig, ESRF 1998).
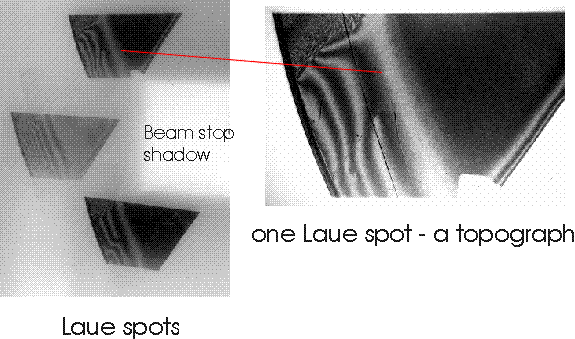
Figure 5: White beam topograph of a SIMOX wafer. Dislocations, scratches, Moiré fringes, and Pendellösung (equal thickness) fringes are visible.
The white beam technique exhibits advantages and drawbacks:
Advantages of White Beam Topography
-
- simplest technique
- no sample orientation necessary (sample environment, growing crystal, …)
- several reflections simultaneously (contrast extinction studies, crystal orientation)
- all crystal parts visible simultaneously (also strongly misoriented or bent ones)
- high diffracted intensity (dynamical experiments, video recording)
- high geometrical resolution
Drawbacks of White Beam Topography
-
- heat load sensibility
- higher harmonics "contamination"
- limited sensibility to weak distortions (see e.g. fig. 7)
b) Lang (projection) topography
The X-ray topographical technique qualitatively similar to the white beam topography and mostly used in laboratories is the "Lang technique" [7]. To help users to compare their results obtained in the laboratory with those obtained at the synchrotron, we will add a short comparison of these two imaging techniques.
From the image formation point of view Lang topography is a extended beam (realised by scanning a rather large beam over the crystal), integrated wave technique. The image recorded on the detector is an integration over a spatial distribution of line sources (divergent waves) on the entrance surface of the crystal, that is a distribution of monochromatic cylinder waves, or in other words a superposition of a spatial distribution of section topographs (see below).
The basic condition for an integrated wave technique d>whQ is fulfilled without problems for laboratory sources with an angular source size d in the order of 10-3 and whQ in the order of 10-5. In addition, laboratory sources are mostly far from being monochromatic. Their wavelength spread Dls of about 3× 10-4×l, is very often much larger than whl. Also in this case this results in second, redundant integration of the same character. However, because of the large d, and consequently the reduced geometrical resolution r, the sample to detector distance should be kept as small as possible.
Important consequences of the above mentioned properties of the Lang technique are:
-
- crystal parts with effective misorientation dQ B (see below) smaller than d are visible simultaneously,
- these parts look identical like in white beam topographs (apart from possible higher harmonic contributions),
- crystal parts with larger effective misorientation are out of contrast.
2.1.2 Double crystal topography
To enhance strain sensitivity and to suppress background from radiation not used in the image formation, more than one crystal should be used in topographic set-ups. The possible two- or more-crystal arrangements in topography are the same like in diffractometry or spectroscopy. Basically there exist two important groups - non-dispersive and dispersive settings(figs.6).
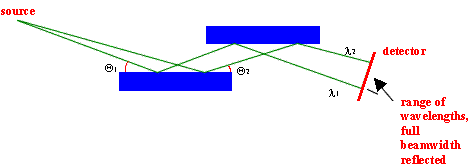
Figure 6 a: Non-dispersive setting ( (+n,-n) setting). The two crystals are identical and the same reflection is used on both. The diffraction vectors are anti-parallel.
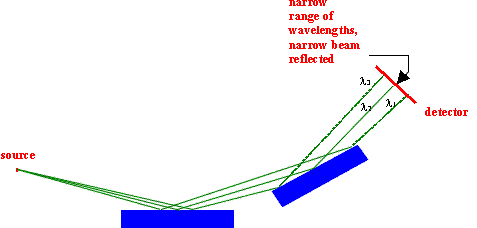
Figure 6 b: Dispersive setting ( (+n,+m) setting). The two crystals may be different and different reflections may be used on both. The angle between the diffraction vectors may be smaller (this figure) and larger than 90 degrees.
One special variant of double crystal topographic techniques with a non-dispersive setting (lattice planes of collimator and sample crystal parallel with the same lattice plane distances) is plane wave topography with d << whQ. With this technique very high strain sensitivities may be achieved. Another variant is weak beam topography. There one works far away down on the flanks of the rocking curve, that is the sample is set far from the Bragg peak. In this way it is possible to detect with high spatial resolution (the limit given by the used detectors is about 1 mm) more strongly deformed regions, like for example regions closer to the centre of a dislocation.
An important practical question is that of the dimension of the image on the detector. For a source not very distant from the sample and a detector very close to it (laboratory case), and using symmetrical reflections the image dimension in the scattering plane is practically equal to that of the source and every part of a topograph is created by the same wavelength (but coming from different places of the source). A possibility to change this dimension is to use asymmetric reflections (increase and reduction) or to use large source to sample distances (increase; synchrotron case). To obtain image dimension perpendicular to the scattering planelarger than the source size is possible by increasing the source to sample distance (laboratory and synchrotron case). Consequently, small and distant sources illuminate the different parts of the crystal (in and perpendicular to the scattering plane) with slightly different angles. The consequences are more crucial for the situation in the scattering planes, therefore it will be discussed in more detail.
Due to the different angles the source shines on different crystal parts, the Bragg condition is fulfilled there for different wavelengths. For a non-dispersive setting the second crystal simultaneously fulfils the Bragg condition for all these wavelengths. Consequently, the image (topograph) may be considerably larger than the source size (fig. 6 a) also within the diffraction plane. Its size is limited in both directions only by the beam divergence, which is considerably larger than d (in the order of 20 arcsec instead of 0.2 arcsec for ID19). As already mentioned, the "prize" for that enlargement is a change of wavelength (energy) along the image in the scattering plane and perpendicular to it. This changing wavelength in the topograph has practically no influence on the image contrasts.
This situation dramatically changes in the case of a dispersive setting. Now the second crystal fulfils the Bragg condition (in the dispersion plane) only in a narrow band (fig. 6 b). Consequently, the diffracted beam and with that the image stay large in the direction perpendicular to the diffraction plane, but becomes narrow in that plane.
What concerns the application for topography, dispersive settings with laboratory sources are not commonly used, because they limit the spatial resolution and the strain sensitivity (due to the large spectral width Dls and consequently a wide reflectivity curve with shallow flanks). These limitations may be reduced by decreasing the spectral width e.g. by a collimator-monochromator-combination like that of DuMond-Bartels.
With synchrotron sources the situation changes considerably. The small angular size of the source and one crystal limit the spectral window to about
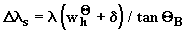 , what is very close to the value w h l of this crystal. In this way we have produced with only one crystal a situation which can only be realised in the laboratory with a double crystal setting. A problem is the above mentioned narrow reflecting band of the crystal in the scattering plane and consequently the narrow image. This problem may be overcome by a slight bending of the first crystal (monochromator), in the same manner as it is used for compensating sample bending. In this way, at synchrotron sources the non-dispersive and dispersive settings give almost the same results concerning strain sensitivity and dimension of the reflecting crystal region. The gain in strain sensitivity when going from white beam to double crystal topography is demonstrated in figure 7 (J. Härtwig, W. Ludwig, ESRF 1997).
, what is very close to the value w h l of this crystal. In this way we have produced with only one crystal a situation which can only be realised in the laboratory with a double crystal setting. A problem is the above mentioned narrow reflecting band of the crystal in the scattering plane and consequently the narrow image. This problem may be overcome by a slight bending of the first crystal (monochromator), in the same manner as it is used for compensating sample bending. In this way, at synchrotron sources the non-dispersive and dispersive settings give almost the same results concerning strain sensitivity and dimension of the reflecting crystal region. The gain in strain sensitivity when going from white beam to double crystal topography is demonstrated in figure 7 (J. Härtwig, W. Ludwig, ESRF 1997).
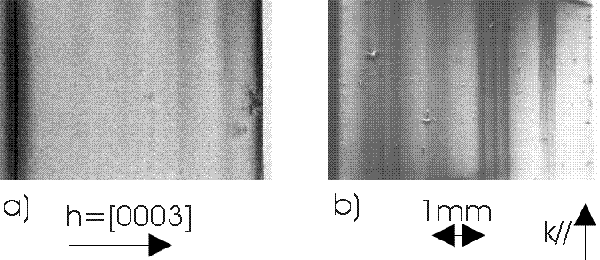
Figure 7:Topographs of a quartz sample with induced growth striations (Y-cut plate, about 1 mm thick [8]). a) white beam topograph, 0003-reflection, b) double crystal topograph, symmetrical 8000-Bragg-reflection (whQ= 0.07 arcsec), high angle flank of rocking curve, bent Si monochromator, asymmetrical 448-Bragg-reflection (whQ= 0.31 arcsec), E = 17.7 keV. The striations are two times two groups with maximum relative lattice parameter changes Dd/d of about 2.5 10-7 and 4 10-7.
In addition to the correction of dispersion, a bent monochromator (or collimator) may be used to correct a bending of the samples. This is often necessary e.g. for wafers with superposed layers, commonly used for microelectronical applications. Figure 8 shows the principle, and figure 9 an example (courtesy R. Köhler, Berlin).
Figure 8: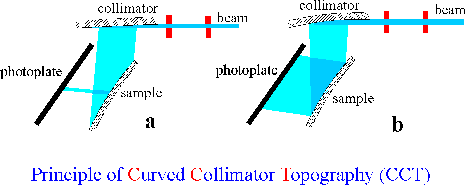
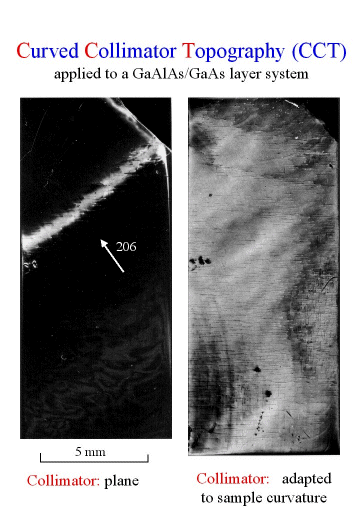
Figure 9: Example of a compensation of sample bending by a curvable monochromator/collimator [9].
The local X-ray contrast in double crystal topographs may be qualitatively explained in an easy way by shifting the angular position of the working point with the effective misorientation dQ (see below)
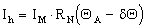 ,
,
where IM is the maximum intensity, RN the normalised reflectivity curve, and Q A the Bragg angle for a (perfect) reference region [10]. Figures 10 and 11 show an example [11].

Fig. 10: Bragg case plane wave topograph of a quartz plate with non-homogenous distributions of impurity atoms, symmetrical ( ) reflection, E = 8.04778 keV, kox is the projection of the wave vector of the incident wave on the crystal surface, working point of about 30% of the maximum intensity on the high angle side of the rocking curve, A, A’, B, B’ denote positions of four step-like changes of impurity concentration.
) reflection, E = 8.04778 keV, kox is the projection of the wave vector of the incident wave on the crystal surface, working point of about 30% of the maximum intensity on the high angle side of the rocking curve, A, A’, B, B’ denote positions of four step-like changes of impurity concentration.
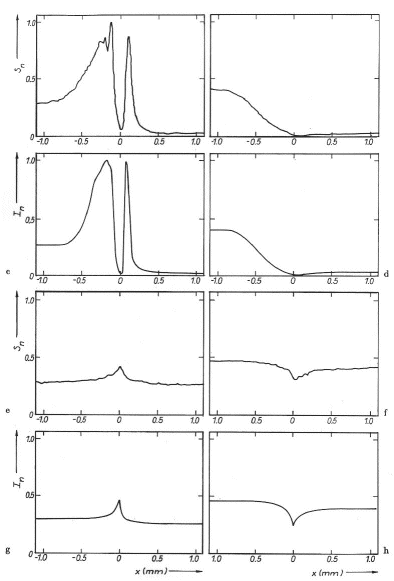
Fig. 11: Comparison of measured (a, b, e, f) photographic density distributions (Sh) corresponding to fig. 10, with calculated (c, d, g, h) intensity distributions (Ih). Related step heights in relative lattice parameter changes: steps A, A’ - 1.12 10-5 (a-d) and B, B’ - 3 10-7 (e-h).
2.2 Section topography (limited beam method)
If we restrict the width of the incoming beam perpendicular to the scattering plane, to a few micrometers (typically 10 - 20 mm), then we obtain after diffraction from a perfect crystal an inhomogeneous intensity distribution in the topograph. In the low absorption Laue case and the Bragg case we obtain a characteristic pattern of interference fringes, and in the high absorption Laue case one fringe in the centre of the image (fig. 12). This pattern is changed sensitively by strain fields. This works either with a white and parallel or a monochromatic and divergent beam.
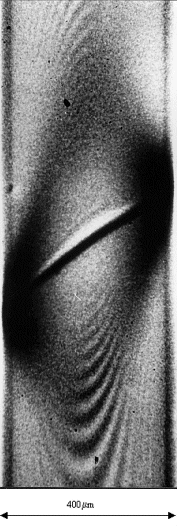
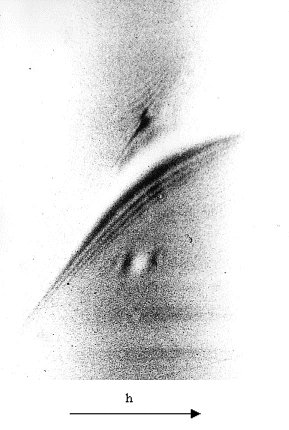
Fig. 12: Section topographs in transmission of a quartz plate (same as in fig. 10, "step" A),  reflection, E = 17.48 keV (left), E = 8.048 keV (right)
reflection, E = 17.48 keV (left), E = 8.048 keV (right)
Is possible to be provide a general quantitative criterion for the necessary beam width, but it is not a simple formula, because we have to take into consideration the diffraction geometry, crystal and source parameters.
Like plane wave topographs, section topographs are rather easy to simulate. By comparison of theoretical with experimental images it is possible to quantitatively describe the investigated strain fields (fig. 13). In addition these methods are also very sensitive to weak deformations.
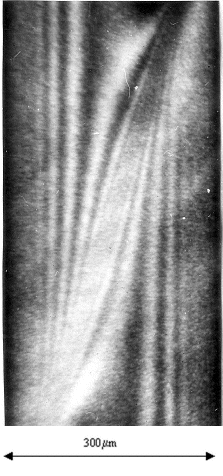

Figure 13: Comparison of a measured (left) and a calculated (right) section topograph, silicon crystal with silicon oxide film edges, film thickness 150 nm, plate thickness 565 mm, symmetrical 422-reflection, E = 17.48 keV [12].
Section topography may also be applied to samples which exhibit regions with strong deformation. Using the positions of direct images (Laue case, low absorption; see below) in a section topograph with respect to the direction of the diffraction vector, it is possible to obtain information about the depth of the related defects in the crystal (fig. 14). This is a mean to determine e.g. a defect distribution with depth. This depth information is normally lost during the "projection of the 3-dimensional strain fields" on the 2-dimensional detector. An alternative is to use stereo pairs of reflections.
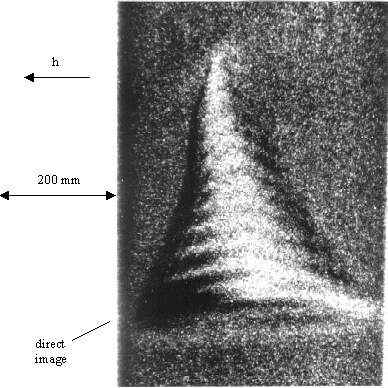
Figure 14: Image of a dislocation in a quartz plate. The direct beam intersects the dislocation line close to the entrance surface, plate thickness 1.8 mm, (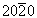 )-reflection, E = 22.163 keV (J. Härtwig, Jena, 1978).
)-reflection, E = 22.163 keV (J. Härtwig, Jena, 1978).
The rarely applied technique using an incoming beam restricted to several micrometers in two perpendicular directions is called pinhole topography.
A short summary of the advantages of synchrotron radiation sources for diffraction topography is given below:
Intensity: exposure times may be (sub) seconds
dynamic experiments, weak reflections,
weak beam technique, thin layers
Wavelength range: white beam Laue pattern
several images simultaneously
high energies accessible
Tunability: choice of wavelength
best information, absorption edges
Polarisation: choice of polarisation
control rocking curve width
(vary strain sensitivity and penetration depth independently)
Time structure: stroboscopic diffraction imaging
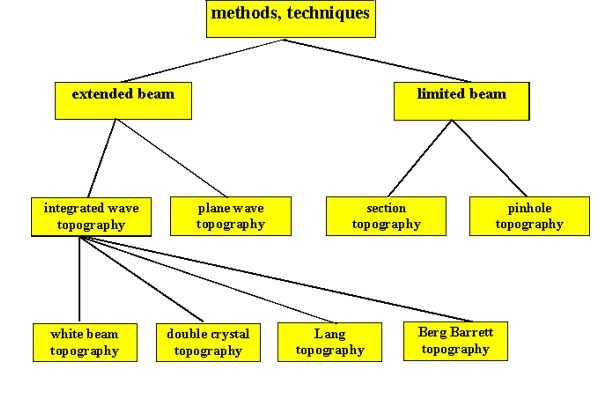
A scheme of the basic topographical techniques is given below:
There are three fundamental mechanisms for contrast in X-ray topographs.
a) Structure factor contrast
The intensity diffracted by a crystal is among other parameters basically a function of the structure factor Fh (which enters the diffraction theories via the Fourier components of the electric susceptibility ch). Thus, regions with different Fh should diffract different intensities. In the case of X-ray this appears e.g. for twinned crystals or ferroelectric domains (fig. 15).
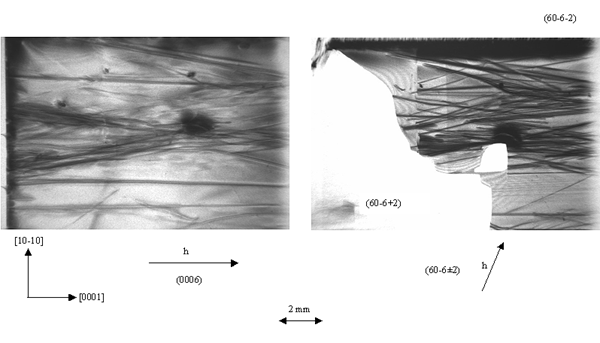
Figure 15: White beam topographs of a quartz plate with twins showing structure factor contrast for reflections h k i ±l . Straight lines are dislocation images.
b) Orientation contrast
If part of a crystal is misoriented in such a way that either diffraction cannot occur at the same time as from the rest of the crystal, or that the diffracted beam from the two parts (assumed to be rather perfect) of the crystal make different directions in space, this is called orientation contrast. It occurs on a macroscopic (global) scale, e.g. for subgrain boundaries, domains, … (fig. 16a, b), but also at a microscopic (local) one, e.g. around lattice defects like dislocations (fig. 17). Its appearance is different depending on the relation between the value of the misorientation (more exactly - the effective misorientation which may arise from dilatations as well as rotations of the lattice) and the spectral and/or angular width of the incoming beam. In addition it may depend on the dimension of the misoriented region, if it has dimensions smaller or in the order of the Pendellösung length (see following section, extinction contrast, direct image), which is a characteristic length parameter for diffraction phenomena in rather perfect crystals:
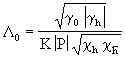 ,
,
where K=1\l is the wave number in vacuum.
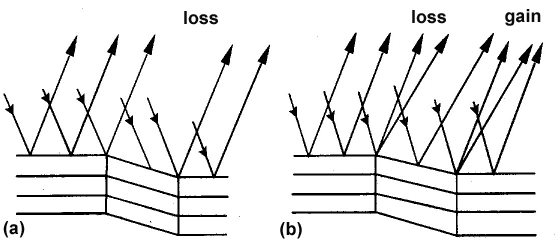
Figure 16a: Schematic diagram of the origin of orientation contrast. The misorientation is a) larger than (no diffraction in the misoriented part) b) smaller than d or Dlstan(QB)/l (misoriented part diffracts, but in a different direction) [2].
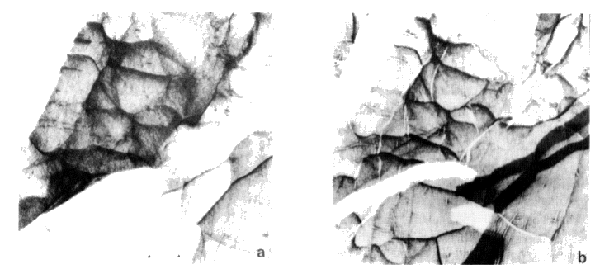
Figure 16 b: Example for Bragg reflections from a LiF crystal with subgrains. a) Lang topograph (left side), b) white beam topograph [13].

Figure 17: White beam topograph in reflection geometry of SiC crystal plate showing the outcrops of micropipes mainly due to a local misorientation contrast. Q B » 80°, 0 0 0 24 - reflection for E » 10 keV, and 0 0 0 30 - reflection for E » 12.5 keV.
c) Extinction contrast
The above two kinds of contrast result from simple considerations like Bragg’s law or general scattering properties. The third type of contrast is related to the local changes of the scattering power, e.g. around a defect. To interpret such changes in detail, it is necessary to use results of the dynamical diffraction theory for perfect and/or for deformed crystals.
Despite the complexity of the contrast formation, one basically parameter controls the process in all variants of the dynamical diffraction theory for a deformed crystal - the effective misorientation:
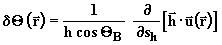 , and/or in some cases its derivative, the strain gradient:
, and/or in some cases its derivative, the strain gradient:
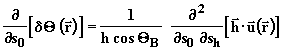 ,
,
where  is the displacement vector and s0 and sh are the directions of the forward diffracted and diffracted beams, respectively.
is the displacement vector and s0 and sh are the directions of the forward diffracted and diffracted beams, respectively.
The effective misorientation is very helpful for the qualitative interpretation of contrasts. Defining locally the dilatation and rotation (relative change of the lattice parameter d d/d and inclination angle of the reflecting lattice planes d j with respect to the perfect lattice) of the lattice it is possible to produce a more "transparent" form of 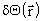 :
:
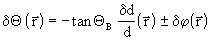 ,
,
where the double sign has to be chosen taking into account if the Bragg angle is locally increased or decreased by the deformation.
The mentioned three mechanisms are the fundamental ones and it is of course possible to subdivide them. We will mention only one important special type of extinction contrast. This is the direct image (additional intensity!) originating from regions with rather big effective misorientation (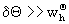 ), e.g. around a dislocation (e.g. in fig. 14 and 15). It appears under the conditions:
), e.g. around a dislocation (e.g. in fig. 14 and 15). It appears under the conditions:
1. of integrated wave topography or section topography,
2. of low absorption, and
3. that the effective misorientation around the defect changes locally by an amount of at least
whQ over distances smaller than L0.
Direct images from dislocations or "spherical" defects are very often seen in practice.
On a given topograph contrasts associated with all the mentioned contrast mechanisms may appear simultaneously. Transitions between them, especially between orientation and extinction contrasts, may be continuous. An experimental proof for the contribution of extinction contrast in relation to misorientation contrast is to record topographs with a sample to detector distance close to zero. Under these conditions (and good geometrical resolution) the misorientation contrast disappears, leading to a homogeneous intensity distribution in the absence of extinction contrast.
4. X-ray topography on thin layers
All the mentioned techniques may be applied to the thick substrate material of a layer system, but for the layers themselves the limitations are different.
Comparing generally the possibilities of topographical methods for the characterisation of thin layers with those of diffractometric methods, one could come to the conclusion that the first ones are much less powerful and apply to much thicker layers.
In X-ray diffractometry one may investigate very thin layers, using its periodic (superlattices) or pseudo-periodic structure (systems of thin layers). but on the cost of a reduced or lost spatial (lateral) resolution (or obtaining only averaged parameters). In addition in those measurements variations of the reflected intensity of up to seven orders of magnitude are currently used.
For the topographical methods an important general constraint is that a defect is usually a non-periodic, localised object. It only disturbs locally the perfect periodic order. Taking into consideration the weak interaction of X-rays with matter, the diffracting defect volume (or volume of the strain field) may not be decreased to any minimum dimension. To detect a non-periodic object, a minimum volume of matter is necessary. This is to see in relation with a second constraint. The necessary contrast modulation to detect a defect on one topograph is usually in the order of some percents. This is several orders of magnitude less than the variation of reflected intensity in a diffraction measurement. One way to detect weaker contrast modulations is to use many-crystal set-ups and to suppress background radiation to a maximum amount possible.
After these general aspects the special properties of X-ray methods in characterising thin layers will be discussed.
a) Integrated wave topography
Orientation contrast from very thin layers, much thinner than the Pendellösung length L0, may be observed when using, as it is possible at ID19, a big enough sample to detector distance. This allows to study for instance their bending.
However, extinction contrast originating from narrow regions with rather big effective misorientation, e.g. around a dislocation within the layer, and creating a direct image, may be observed only if the layer thickness is not smaller than about 0.15×L0××× 0.4×L0. The physical reason for that fact is that the integrated diffracted intensity does anymore differ for deformed and perfect parts if the crystal is very thin.
b) Double crystal topography
This limitation concerning the visibility of regions with rather big effective misorientation holds for integrated wave techniques, but is not valid in the case of double crystal techniques which exhibit narrow wavelength or/and angular bandpasses. In this last case the contrast behaviour is controlled straightforward by the effective misorientation.
c) Moiré topography
If a layer is situated on a thick substrate and their lattice parameters difference is so small that they diffract simultaneously, then the wave fields of both crystals may interfere. This interference effect, called Moiré effect, enhances considerably the sensitivity to weak strains and the visibility of localised defects like e.g. threading dislocations. In this way it is for example possible to investigate thin layers on thick perfect substrates with rather high energies and in the transmission (Laue) case.
The detection limit to small deformations depends among others on the nature of these deformations. For defects like growth striations it is roughly in the order of 10-6 for the integrated beam techniques. This sensitivity may be improved by one to two orders of magnitude by double crystal topography or even slightly more by exploiting interferometric effects (Moiré topography).
Concerning layer thickness, it is rather difficult to detect defects and to measure the homogeneous deformation in layers made from light elements like silicon, if the layers are much thinner than about 1 m m and if using the mentioned standard techniques. The heavier the elements, the thinner the layers may be (see example in fig. 18, A. Remhof, G. Song, D. Labergerie, J. Härtwig, H. Zabel, Bochum & Grenoble: ESRF 1998).
In all cases we have considerable possibilities to shift the mentioned limits by changing diffraction parameters and geometry (wavelength, reflection, order of reflection, asymmetry, … ). Going beyond the above mentioned rough "limits" is the task of "extreme" techniques like topography in grazing incidence or others.

Figure 18 : 110 Bragg reflection of a 50 nm thick niobium layer. l = 0.07 nm, E = 17.7 keV.
5. New possibilities of modern synchrotrons
The range of applications of the topographic techniques grew considerably when synchrotron radiation was available. They could be applied to study under reasonable conditions very weak reflections, weak distortions, in-situ crystal growth, defect nucleation and movement in crystals and thin films, dynamics of domains, phase transitions... . The use of the new features of third generation synchrotron radiation machines results in another jump. Some of these features (high intensity, high energy photons in the beam) extended substantially the previous possibilities (investigation of heavier or thicker crystals, study of dynamical phenomena with smaller typical times) and enhanced the spatial resolution (weak beam technique, systematic use of section topographs).
The small angular size of the source d leads to a very high geometrical resolution and a large transversal coherence length. It allows to use as a new parameter the propagation distance of the X-rays after leaving the sample. This propagation leads to considerably enhanced effects, e.g. the separation of misoriented regions in topographs (at ESRF ID19, one may record topographs with film to sample distances of more than one meter without considerable loss of resolution), and to new effects like focusing (or defocusing) by defects, or phase contrast images.
[1] X-ray Diffraction Topography by B. K. Tanner, , Pergamon Press 1976
[2] X-ray and Neutron Dynamical Diffraction edited by A. Authier, S. Lagomarsino,
B. K. Tanner, Plenum Press 1996
[3] Bowen Tanner
[4] G. N. Ramachandran, X-ray topographs of diamond, Proc. Indian Acad. Sci., Sect. A, 19,
280 (1945)
[5] M. Schlenker, J. Baruchel, R. Perrier de la Bâthie, Neutron-diffraction section topography:
Observing crystal slices before cutting them, J. Appl. Phys. 46, 2845-48 (1975)
[6] T. Tuomi, K. Naukkarinen, P. Rabe, Use of synchrotron radiation in X-ray diffraction
topography, Phys. Stat. Sol. (a) 25, 93-106 (1974)
[7] A.R. Lang, The projection topograph: A new method in X-ray diffraction
microradiography, Acta Cryst. 12, 249 (1959)
[8] U. Alter, J. Härtwig, X-ray topographic study of quartz crystals with induced growth
striations, Cryst. Res. Technol. 20, 1173-80 (1985)
[9] B. Jenichen, R. Köhler, W. Möhling, Double crystal topography compensating for the
strain in processed samples, Phys. Stat. Sol. (a) 89, 79 (1985)
[10] U. Alter, J. Härtwig, J. Kubena, Plane wave topography on crystals with step-like
impurity distributions, Czech. J. Phys. B 35, 158-167 (1985)
[11] J. Härtwig, V. Holý, R. Kittner, J. Kubena, V. Lerche, Investigation of quartz crystals
with non-homogeneous distributions of impurity Atoms by X-ray methods,
Phys. Stat. Sol. (a) 105, 61-75 (1988)
[12] J. Härtwig, H.-J. Witt, J. Bak-Misiuk, Representation of simulated X-ray topographs by
means of the FEAG 200 device, Cryst. Res. Technol. 33, 1417-24 (1988)
[13] M. Hart, J. Appl. Cryst. 8, 436 (1975)
Jürgen Härtwig, February 1999



