Competition between different ground states is a central issue in condensed-matter physics. Much discussed examples are those between superconductivity and magnetism in cuprates, iron pnictides, and heavy fermions. Equally important is that between a charge density wave (CDW) and superconductivity. We have employed inelastic X-ray scattering (IXS) and ab initio calculations to investigate the mechanisms that govern such an interplay in uranium.

Uranium crystallises at room temperature in an unusual orthorhombic structure (α-U phase) unique for an element at ambient pressure. A phase transition to a CDW state occurs below 43 K [1] and this behaviour has been ascribed to nesting of certain features of the Fermi surface [2]. The CDW state (called α1-U), to a first approximation, may be considered as a doubling of the a-axis of the unit cell. Under pressure the CDW disappears at around 1.5 GPa while superconductivity exhibits a maximum transition temperature (Tc) of about 2 K.

The key aspect, which stabilises the low-symmetry α-U structure is the narrow band containing about three 5f electrons at the Fermi level. Treating accordingly the 5f electrons, the unusual phonon spectrum of α-U was reproduced only in 2008 by ab initio calculations [3], almost 30 years after its experimental determination [4]. A prediction of this calculation is that, under pressure, the energy of the soft phonon mode Σ4 in the [100] direction increases, until the anomaly disappears near 20 GPa.

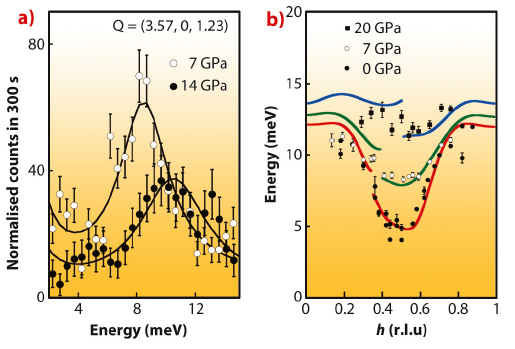
To validate this theoretical prediction we performed an IXS experiment at beamline ID28 on a single-crystal sample placed in a diamond anvil cell. Figure 14a shows IXS spectra taken around the key position in reciprocal space for two pressures. This establishes the hardening of the Σ4 mode as pressure is increased. Figure 14b shows the experimental (points) and theoretical (lines) dispersion along the [100] direction for three pressures.

Fig. 14: a) IXS phonon spectra of α-U for Q = (3.57, 0, 1.23) at 7 and 14 GPa, and T = 297 K b) Measured (symbols) and calculated (lines) phonon dispersion of the Σ4 mode in α-U at the three indicated pressures.

The excellent agreement between theory and experiment led us to further investigate the phase diagram. Our theoretical studies reveal that the Fermi surface, and consequently the nesting conditions, evolves only very little with pressure. In contrast, we found that the electron-phonon coupling is very sensitive to the wave-vector, reaching a maximum where the soft mode occurs. This maximum is, however, rapidly suppressed when pressure increases. Thus, electron-phonon coupling is the crucial ingredient for the formation and the disappearance of the α1-U state. Fermi-surface nesting is indeed present, but although necessary, it is not the unique ingredient.

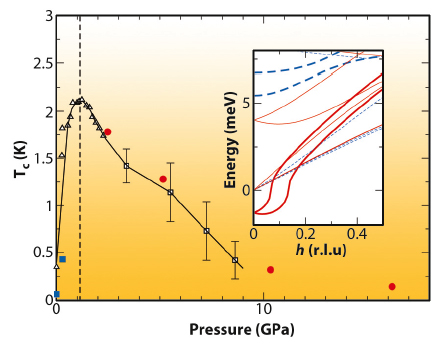
For phonon-mediated superconductivity, the knowledge of the electron-phonon coupling allows, furthermore, the determination of Tc. Our calculation shows that electron-phonon coupling increases in the CDW phase until the structure is unstable just above 1 GPa (see inset of Figure 15). In contrast, at higher pressures the electron-phonon coupling decreases in the a phase, as discussed above. Thus the dome shape of the superconducting region is explained by the pressure dependence of electron-phonon coupling in concomitance with the respective stability of the α1-U and α-U phases. Figure 15 shows the good agreement between the measured and calculated superconducting transition temperatures as a function of pressure.

Fig. 15: T-P phase diagram of uranium in the superconducting region. The vertical line indicates the calculated transition between α1-U and α-U phases. The experimental data (open triangles and squares) for Tc are taken from Ref. [1]. The blue squares and red circles correspond to the calculated Tc in the α1-U and α-U phases, respectively. The inset shows the phonon dispersion in the α1-U phase at 0 (blue) and 5 (red) GPa.

 

Our phonon measurement in uranium confirms the theoretically predicted disappearance of the soft mode under pressure. Further investigation of the mechanism at play emphasises that the momentum and pressure dependence of the electron-phonon coupling plays the central role in determining the complex phase diagram of uranium including the CDW and the superconductivity, whereas the Fermi-surface nesting is independent of pressure.

 

Principal publication and authors

S. Raymond (a), J. Bouchet (b), G.H. Lander (c), M. Le Tacon (d,e), G. Garbarino (d), M. Hoesch (d), J.-P. Rueff (f,g), M. Krisch (d), J.C. Lashley (h), R.K. Schulze (h) and R.C. Albers (h), Physical Review Letters 107, 136401 (2011).

(a) CEA, DSM, INAC, Grenoble (France)

(b) CEA, DAM, DIF, Arpajon, (France)

(c) Institute for Transuranium Elements, Karlsruhe (Germany)

(d) ESRF

(e) Max-Planck-Institut für Festkörperforschung, Stuttgart (Germany)

(f) Synchrotron SOLEIL, Gif-sur-Yvette (France)

(g) Laboratoire de Chimie Physique, Paris (France)

(h) Los Alamos National Laboratory, New Mexico (USA)

 

References

[1] G.H. Lander et al., Advances in Physics 43, 1 (1994) and references therein.

[2] L. Fast et al., Phys. Rev. Lett. 81, 2978 (1998).

[3] J. Bouchet, Phys. Rev. B 77, 024113 (2008).

[4] W.P. Crummett et al., Phys. Rev. B 19, 6028 (1979).