- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2009
- Enabling Technologies
- Focusing monochromator with extreme energy resolution
Focusing monochromator with extreme energy resolution
High energy resolution is a figure of merit for many synchrotron radiation techniques. The most extreme requests come from inelastic X-ray scattering and nuclear inelastic scattering, where ~1 meV and sub-meV resolution is reached. An even higher energy resolution is needed for demanding scientific cases such as the novel high-TC superconductors, liquids, and biological materials. Therefore, the improvement of the energy resolution down to 0.1 meV is a key project at several synchrotron radiation facilities.
Attempts to reach an energy resolution of 0.1 meV with conventional optical schemes encounter severe losses of intensity [1,2]. Analysing alternative schemes with higher throughput, we propose an approach with a ‘focusing monochromator’, whereby the X-ray energy spectrum is swept along the spatial coordinate rather than along the angular coordinate.
 |
|
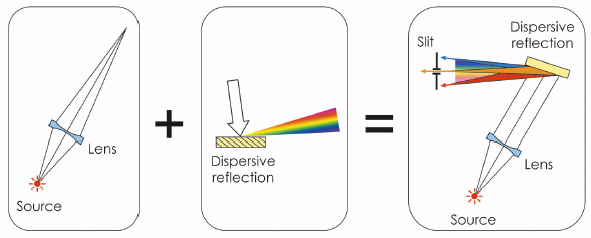
Fig. 146: The concept of a ‘focusing monochromator’: By the combined action of the focusing lens and the dispersive crystal, X-rays of different energies are focused to different focal spots. Selection of the narrow energy band is achieved by the slit. |
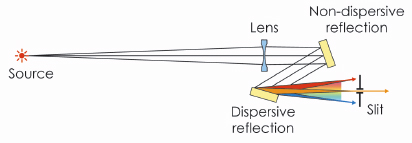
The operation of the focusing monochromator is based on a combined action of a focusing lens and a dispersive crystal (Figure 146). The lens alone would focus X-rays of all relevant energies towards a single spot. The crystal in a highly asymmetric reflection works as an optical prism [3], sorting the X-rays of different energies into different directions. In the combined action, the lens focuses X-rays through the dispersive crystal. Deflecting the X-ray components of different energies by different angles, the crystal re-directs them to different focal spots. The selection of the narrow energy band is provided by a slit located in the focal plane. The more convenient in-line geometry of the monochromator is achieved by adding another crystal with the same reflection, but in a symmetrical scattering geometry (Figure 147).
The ultimate relative energy resolution ![]() E/E of the focusing monochromator is determined by the angular size of the radiation source as
E/E of the focusing monochromator is determined by the angular size of the radiation source as
![]()
where ![]()
![]() = S/L is the angular size of the radiation source as seen from the lens location; S is the spatial source size; L is the source-lens distance; b is the module of the reflection asymmetry parameter; and
= S/L is the angular size of the radiation source as seen from the lens location; S is the spatial source size; L is the source-lens distance; b is the module of the reflection asymmetry parameter; and ![]() B is the Bragg angle. This resolution is reached with the slit size equal to the focal spot of monochromatic radiation. Opening the slit, one can gradually increase the bandwidth of the selected radiation up to the entire energy band reflected by the crystal. This offers a useful possibility to perform fast measurements with coarse resolution but high count rate before studying selected features with the ultimate energy resolution.
B is the Bragg angle. This resolution is reached with the slit size equal to the focal spot of monochromatic radiation. Opening the slit, one can gradually increase the bandwidth of the selected radiation up to the entire energy band reflected by the crystal. This offers a useful possibility to perform fast measurements with coarse resolution but high count rate before studying selected features with the ultimate energy resolution.
For a moderately high asymmetry parameter b, the relative energy resolution ![]() E/E is determined only by the angular size
E/E is determined only by the angular size ![]()
![]() and the Bragg angle
and the Bragg angle ![]() B . The smallest angular size of the radiation source available at the ESRF (for a 200 m-long beamline) is 0.1 µrad. Then, the 0.1 meV bandwidth can be obtained with a Bragg angle of 84 or 87 degrees for X-rays with an energy of 10 keV or 20 keV, respectively.
B . The smallest angular size of the radiation source available at the ESRF (for a 200 m-long beamline) is 0.1 µrad. Then, the 0.1 meV bandwidth can be obtained with a Bragg angle of 84 or 87 degrees for X-rays with an energy of 10 keV or 20 keV, respectively.
 |
|
Fig. 147: In-line geometry of the focusing monochromator with an additional crystal in symmetric reflection. |
The proposed optical scheme is expected to provide very high throughput of radiation within the selected energy band. According to the operation requirements (see principle publication), the lens delivers a highly collimated beam well within the angular acceptances of both crystals. In addition, the moderate asymmetry parameter allows one to keep the high reflection coefficient.
From a practical point of view, the proposed design is simple and includes a minimal number of optical elements. Relative to conventional high-resolution optics, the X-ray spot on the crystals of the focusing monochromator is much smaller, well below 10 mm. Therefore, the proposed design should be relatively insensitive to possible imperfections of the crystal quality.
References
[1] M. Yabashi, K. Tamasaku, S. Kikuta and T. Ishikawa, Rev. Sci. Instrum. 72, 4080 (2001).
[2] T. S. Toellner, M. Y. Hu, W. Sturhahn, G. Borel, E. E. Alp and J. Zhao, J. Synchrotron Rad. 8, 1082 (2001).
[3] Yu. Shvyd’ko, X-Ray Optics, Springer-Verlag Berlin Heidelberg, 2004.
Principal publication and authors
V. G. Kohn (a), A. I. Chumakov (b) and R. Rüffer (b), J. Synchrotron Rad. 16, 635 (2009).
(a) Russian research center ”Kurchatov Institute” (Russia)
(b) ESRF



