- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2000
- Electronic States and Lattice Dynamics
- Determination of the Short-wavelength Propagation-threshold in the Collective Excitations of Liquid Ammonia
Determination of the Short-wavelength Propagation-threshold in the Collective Excitations of Liquid Ammonia
The advent of Inelastic X-ray Scattering (IXS) spectroscopy with meV energy resolution has widened the possibility of studying collective atomic dynamics in disordered systems, such as liquids and glasses, in the momentum transfer (Q) region approaching the inverse of the inter-particle separation, Q0. A common feature in studies of glasses, glass-forming systems and molecular liquids is the observation of propagating collective modes up to a maximum Q-transfer, Qm, which is a relevant fraction of Q0: Q/Qm = 0.1 ÷ 0.5. At Qm the excitation width, ![]() (Q), becomes equal to the excitation energy,
(Q), becomes equal to the excitation energy, ![]() (Q), and therefore the excitation spectrum becomes a featureless broad band. At Q larger than Qm, in the systems studied so far, the increasing elastic component in the S(Q,E) strongly hides the presence of an inelastic signal, often already difficult to detect at Q = Qm. This experimental scenario prompts differences in opinion on how well one can define a propagating collective excitation in the considered high Q-regime. There are in fact different interpretations, which could depend on the specific model chosen to represent the S(Q,E) and to derive the spectroscopic parameter.
(Q), and therefore the excitation spectrum becomes a featureless broad band. At Q larger than Qm, in the systems studied so far, the increasing elastic component in the S(Q,E) strongly hides the presence of an inelastic signal, often already difficult to detect at Q = Qm. This experimental scenario prompts differences in opinion on how well one can define a propagating collective excitation in the considered high Q-regime. There are in fact different interpretations, which could depend on the specific model chosen to represent the S(Q,E) and to derive the spectroscopic parameter.
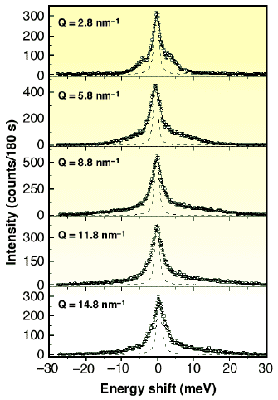 |
Fig. 67: Selected examples of IXS spectra of l-NH 3 taken at the indicated Q values. The IXS data (o) are reported together with their error bars. The dashed lines represent the experimental resolution functions of the five analyser crystals working in parallel. The resolution functions have been aligned and scaled to the quasi-elastic peak. The full line on the three top spectra is the result of the fit to a visco-elastic model performed in the low-Q region. Note the presence of inelastic signal at every Q-value.
|
In the present work, we studied the S(Q,E) of liquid ammonia, l-NH3 - a moderately hydrogen-bonded liquid. In this system, an inelastic signal is observed up to the highest investigated Q value of 15 nm-1, corresponding to Q/Q0 ![]() 0.75. This is shown in Figure 67. In the low Q region, the analysis of the spectra has been performed using the visco-elastic model. This yields results such as the presence of positive dispersion of the sound velocity, which are in line with a similar study on liquid water.
0.75. This is shown in Figure 67. In the low Q region, the analysis of the spectra has been performed using the visco-elastic model. This yields results such as the presence of positive dispersion of the sound velocity, which are in line with a similar study on liquid water.
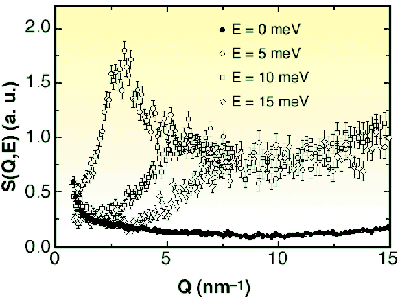 |
Fig. 68: IXS spectra, shown with error bars, taken as a function of Q at the indicated constant energies. The inelastic data (o) have been normalised imposing similar values at Q = 15 nm -11. The elastic spectrum (full symbols) has been normalised to the other spectra in the low Q-region.
|
Most importantly, the presence of a clear inelastic signal in the high Q region, in spite of the absence of a well defined inelastic peak, allows the experimental determination of the S(Q,E) as a function of Q. This has been done for three different values of energy transfer E and the results are reported in Figure 68. The data in Figure 68 show characteristic features such as: i) negligible intensity at low Q, ii) a well defined Brillouin line for small values of E, which gets increasingly broader and then disappears with increasing E, and iii) a Q- and E-independent plateau at Q-values above the Brillouin peak position. The importance of these results is that they provide a qualitative, but model-independent picture, of the short-wavelength dependence of the Brillouin line in a disordered system; specifically, it shows that the observed modes lose their plane-wave character with increasing energy transfer. This qualitative analysis confirms similar results previously obtained on different systems such as simulated glasses. A more quantitative analysis is obtained using the S(Q,E) vs. E spectra such as those reported in Figure 67, and putting them on an absolute scale thus exploiting the general Moments Sum Rules of the S(Q,E). This allows the reconstruction of the S(Q,E) vs. Q at selected constant E-values; this analysis confirms the direct measurements of Figure 68, and, most importantly, it allows us to observe a cross-over from a dynamic regime characterised by the presence of the Brillouin line to one where this feature can no longer be observed; this transition takes place at a cross-over momentum, Qc, which turns out to agree quantitatively with the value, Qm, derived using the visco-elastic model to represent the S(Q,E) of this system.
This experiment has been carried out at the very high-energy resolution IXS beamline ID16. We acknowledge B. Gorges for the construction of the ammonia cell.
Principal Publication and Authors
F. Sette (a), G. Ruocco (b), A. Cunsol (a), C. Masciovecchio (c), G. Monaco (a) and R. Verbeni (a), Phys. Rev. Lett. 84, 4136 (2000).
(a) ESRF
(b) Universitá di L'Aquila (Italy)
(c) Sincrotrone Trieste (Italy)



