- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2006
- High Resolution and Resonance Scattering
- Elasticity of hexagonal BN and graphite
Elasticity of hexagonal BN and graphite
Despite the interest of graphite and hexagonal BN (h-BN) as basic building block for intercalated compounds and nanostructured materials, there is no complete and reliable data set of their elastic properties. There are two reasons for this: Firstly, the lack of sufficiently large single crystals makes the application of classical experimental techniques such as ultrasound measurements impossible; secondly, the spread amongst computational results is large as there are two completely different types of interatomic bonding – exceptionally strong sp2 covalent intralayer bonding and weak van der Waals interlayer bonding.
Inelastic X-ray scattering (IXS) overcomes the intrinsic difficulties of inelastic neutron scattering (INS), namely sample size and energy transfer limitations, and it is not sensitive to the defect structure of the material, unlike ultrasonic methods. Indeed, the first experimental determination of the optical phonons in the entire in-plane Brillouin zone of graphite was performed by IXS [1]. Here we obtained complete sets of the five independent elastic moduli and found the upper limits for the on-axis Young’s moduli of homogeneous nanotubes.
 |
|
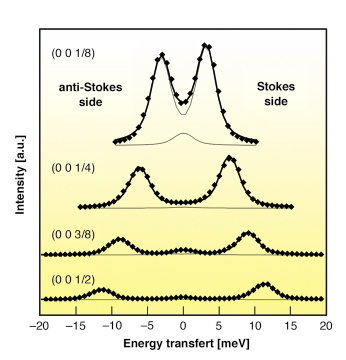
Fig. 14: Longitudinal acoustic phonons of graphite, measured close to the (004) Bragg spot, propagating along the c axis. Reduced momentum transfer values are given in reciprocal lattice units. The experimental data are shown together with the best fit results. The spectra are shifted in the vertical direction for clarity, conserving the same intensity scale. The counting time per point was 40 seconds. |
BN crystals were grown by high-pressure/high-temperature synthesis in a Ba-B-N catalyst system [2], while the graphite crystals were natural. In both cases the samples were platelets consisting of single crystalline domains. The size of a single grain was as large as 0.6-1.0 mm in the lateral direction and 50-120 µm along the c axis. With the small beam dimensions, individual domains with angular spread of 0.025°-0.05° FWHM could be selected. The IXS experiment was performed on beamline ID28. The five independent elastic moduli were obtained via the sound velocities, as derived from the initial slope of the acoustic phonon branches along specific (mostly high-symmetry) directions, and the Christoffel’s equation. The consequence of the high anisotropy of graphite and h-BN is the need to take into account the effect of the finite resolution in momentum transfer q, defined by the angular opening of the analysers. The instrument was operated utilising the silicon (9 9 9) setup, providing a total energy resolution of 3.0 meV. The momentum resolution was set to 0.2 nm-1 and 0.7 nm-1 in the horizontal and vertical plane, respectively. The acquisition time per point varied from 30 to 60 s. An example of the collected data is given in Figure 14.
 |
|
Table 1 : Elastic moduli of hexagonal BN and graphite. |
The resulting elastic moduli (see Table 1) allow us to reconstruct the velocity of sound propagating in any direction as presented in Figure 15. While the overall topology of the sound velocity surface is similar for both systems, the difference in details is quite substantial. The elastic moduli are regularly higher for graphite, except for the C44 value. C44 is much larger in hexagonal BN due to the additional ionic interaction between layers, making them more rigid vs. shear deformation.
 |
|
Fig. 15: 3D plot of the sound velocity data for the h-BN (half-space shown) and its a-c plane section: experimental data are denoted by diamonds, the surface corresponds to the best fit. The low-velocity part is shown enlarged in the bottom panel. |
The values derived from the ultrasonic data are regularly lower than for INS and IXS. This fact is not surprising, as these measurements were performed on pyrolytic graphite and hBN, where the presence of defects greatly influences the macroscopic elastic properties. INS capabilities are limited by the sample size, which imposes again the utilisation of pyrolytic samples. INS measurements of phonon dispersion in graphite were the least reliable for the in-plane longitudinal acoustic branch due to the nature of the sample, while for lower-velocity branches the data are very close to our results.
The elasticity of layered substances is directly linked to the mechanical properties of derived nanotubes, of interest for applications such as high-strength fibres. Our values of C11 = 1.1 TPa for graphite and C11 = 0.8 TPa for h-BN provide the upper estimate of the on-axis Young’s modulus of homogeneous nanotubes. The majority of measurements performed on the nanotubes give overestimated values compared with our evaluations. We believe that our estimates provide a solid background for both theoretical modeling and analysis of experimental data.
References
[1] J. Maultzsch, S. Reich, C. Thomsen, H. Requardt, P. Ordejón, Phys. Rev. Lett. 92, 075501 (2004).
[2] K. Watanabe, T. Taniguchi, H. Kanda, Nature Materials 3, 404 (2004).
Principal Publication and Authors
A. Bosak (a), J. Serrano (a), M. Krisch (a), K. Watanabe (b), T. Taniguchi (b), H. Kanda (b), Phys. Rev. B 73, 041402 (2006); A. Bosak (a), M. Krisch (a), M. Mohr (c), J. Maultzsch (c), C. Thomsen (c), submitted.
(a) ESRF
(b) Advanced Materials Laboratory, National Institute for Materials Science, Tsukuba (Japan)
(c) Institut für Festkörperphysik, TU Berlin (Germany)



