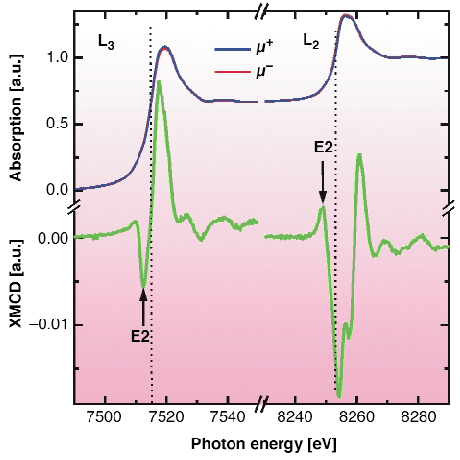
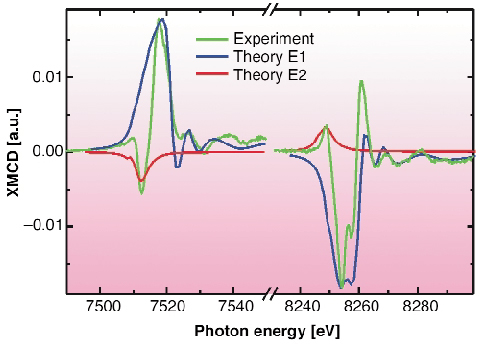
Since the first measurement of X-ray magnetic circular dichroism (XMCD) at the L2,3 edges of rare-earth compounds, extensive series of experiments have been performed. This is due to the great technological importance of these materials, e.g. in high-performance permanent magnets and information storage devices. The dipolar transition (E1: 2p5d) made these spectra appear promising for studies of the role of 5d electrons in the complex magnetic phenomena of the rare-earth materials. Unfortunately, the contribution of the quadrupolar transitions (E2: 2p4f) makes the interpretation of the spectra difficult. Experiments in the past at second-generation synchrotron radiation facilities did not permit measurements of XMCD spectra with detailed fine structure that was free of noise. Only the present performance of the ID12 beamline with the gap-scan technique enables us to detect these fine structures with excellent quality, as shown in Figure 110 [1]. These experimental spectra present a challenge for ab initio theory. Finally, the theory has the advantage of disentangling the E1 and E2 transitions. Heretofore, no simple method has been reported to separate the quadrupolar contributions from the dichroic spectra, since even in angular-dependent XMCD measurements, an overlap of the two contributions is observed. The identification of the quadrupolar contributions in the dichroic spectra is essential for the proper application of the sum rules to determine the magnetic moments. Most of the XMCD data reported in the literature were obtained from rare-earth compounds, which are complicated systems including various many-body interactions. Here we used a single-element Tb crystal with small static disorder, which is very helpful to achieve a fundamental understanding of the rare-earth XMCD signal. The helicity-dependent absorption spectra at 10 K are presented at the top of Figure 110 at both the L3 and the L2 edges and the corresponding XMCD spectra are shown at the bottom. A shoulder peak in the L2 XMCD centred at 5 eV above the absorption edge is observed. This indicates a clear improvement of the quality of XMCD compared to spectra published previously, where this feature could not be detected. In order to disentangle the various fine structures we calculated the XMCD within the local spin density functional approximation by using the most recent version of the real-space multiple scattering code FEFF8 [1]. The calculations presented in Figure 111 demonstrate that the main contributions at the L2 and L3 edges originate from E1 transitions, but the narrow fine structures in the pre-edge regime are due to the E2 transitions. Also the onset of the magnetic EXAFS oscillations is reproduced by the theory [2]. The dipole transition matrix elements are strongly spin-dependent for the rare-earth elements. Therefore, even after separating the E2 and E1 contributions, the results of the sum rule application must be corrected for this effect with the help of the theory. If these corrections are not carried out, an apparent 5d spin moment per atom of µS5d = ­0.27 µB is obtained, and hence erroneously, an antiparallel orientation of the 5d to the 4f moments would be concluded. However, by including the spin dependence of the matrix elements in the sum rule analysis, we obtained µS5d = +0.37 µB, accentuating the need for this correction. This now opens the new and fascinating possibility to use the XMCD technique also for rare-earth elements and compounds with its full strength (element- and shell-specificity, determination of spin and orbital magnetic moments).

 

Fig. 110: Normalised Tb X-ray absorption coefficients for right (µ+) and left (µ­) circularly polarised X-rays (top) and corresponding XMCD spectra (bottom). Quadrupolar transitions (2p4f) are marked with arrows.

 

 

Fig. 111: Comparison of experimental (green) and theoretical XMCD spectra at Tb L2,3 edges: dipolar (blue) and quadrupolar (red) contributions.

 

References
[1] H. Wende et al., J. Appl. Phys., 91, 7361-7363 (2002).
[2] H. Wende et al., J. Synchrotron Rad., 8, 419-421 (2001).

Authors
H. Wende (a), A. Scherz (a), G. Ceballos (a), C. Sorg (a), K. Baberschke (a), A. Ankudinov (b), J.J. Rehr (b), F. Wilhelm (c), A. Rogalev (c), D.L. Schlagel (d), T.A. Lograsso (d).
(a) FUB, Berlin (Germany)
(b) University of Washington, Seattle (USA)
(c) ESRF
(d) Ames Laboratory, Ames (USA)