- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2014
- Dynamics and extreme conditions
- Localisation of propagating phonons in a perfectly crystalline solid
Localisation of propagating phonons in a perfectly crystalline solid
The energy loss due to thermal conversion processes has triggered the search for new functional materials allowing for more efficient energy consumption. Promising candidates are ultra-low thermal conductivity materials that can be made use of in thermoelectric converters for waste heat recovery as well as in thermal barrier coatings. In non-metallic crystalline solids, heat is mainly carried by atomic motion, in the form of propagating waves. In a quantum mechanical picture, the atomic vibrations can be described by means of the free motion of bosonic quasiparticles, known as phonons.
Cage-like structures encapsulating guest atoms or molecules such as clathrates, skutterudites, β-pyrochlore and zeolites are known to exhibit anomalously low thermal conductivities. The encaged atoms were formerly considered as independent oscillators and the observed suppression of the thermal conductivity was then rationalised by considering the inelastic resonant scattering of heat carrying phonons with these oscillators. However, in 2008, a study of phonons in skutterudites demonstrated that this intuitive picture of resonant scattering by loosely bound guest atoms is not applicable, putting into question the current understanding of the anomalous heat properties in these materials [1].
 |
|
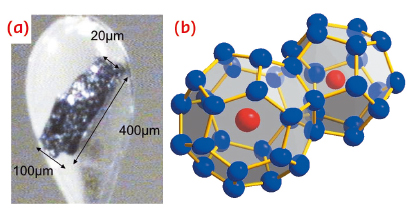
Fig. 75: a) Ba8Si46 microcrystal grown at 5 GPa and 1000°C. b) Representation of face sharing BaSi20 and BaSi24 cages as building units of type-I clathrates. Atoms are represented by their atomic displacement ellipsoids obtained from single crystal X-ray diffraction. |
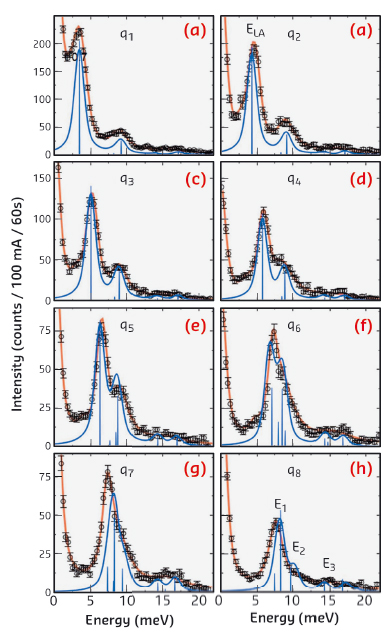
We have investigated the mechanism at the origin of the low thermal conductivity in cage based materials by mapping the phonon states in the simplest representative intermetallic clathrate, Ba8Si46 (see Figure 75), using Inelastic X-ray Scattering (IXS) at beamline ID28. Phonons propagating along the main crystallographic directions were studied and the corresponding IXS cross sections were simulated by ab initio density functional theory (DFT) calculations. In Figure 76, raw data of constant Q-scans, along the [100] direction, are shown together with the corresponding simulated IXS spectra, convoluted with the instrumental resolution function. A surprisingly good agreement between the experimental and the simulated energy profiles can be observed for both the position and the energy width of the phonons. For phonon wave vectors close to the Brillouin zone centre (q –> 0), all phonon spectra consist of a main peak at energies distinctly below the optical (op) guest excitations, which stems from the acoustic (ac) branch and follows a linear dispersion. No energy broadening was needed to fit the acoustic and optic phonon profiles. The main effect that was observed in our experimental data was an abrupt decrease of the intensities of the acoustic phonons for wave vectors higher than a critical wave vector, qc, concomitant with an increase of the intensities of the guest phonons. This was accompanied by a strong bending of the dispersion relation of the acoustic excitations, with its slope approaching zero. qc was interpreted as the limit of the acoustic regime above which the acoustic character of the modes vanishes. The abrupt changes of the acoustic intensities were remarkably well reproduced by the ab initio simulation.
 |
|
Fig. 76: a-h) Example of constant Q-scans for photons propagating along the 001 direction. Orange lines are fits of the data. Blue profiles correspond to the ab initio simulated IXS spectra, convoluted with the instrumental resolution function. The blue vertical lines indicate the simulated phonon positions without the convolution. |
In conclusion, the filling of the cages with guest atoms leads to the suppression of host propagative phonons, which are highly efficient at carrying heat, over a wide range of energies and wave vectors in phase space. The guest-host interaction can be understood as a purely harmonic process, i.e. it is not dominated by phonon-phonon scattering. The acoustic phonons preserve a very long lifetime and their propagative nature, until a critical wave vector is reached: at this point the character of the acoustic phonons changes, resulting in modes with an increasing contribution of the guest atoms. A careful theoretical analysis provides evidence that the guest modes are not propagative. These modes release their energy locally in an isotropic manner, leading to a change from a propagative to a diffusive energy transport mechanism, which may, in turn, be responsible for the low lattice thermal conductivity.
Principal publication and authors
S. Pailhès (a), H. Euchner (b), V.M. Giordano (a,f), R. Debord (a), A. Assy (c), S. Gomès (c), A. Bosak (d), D. Machon (a), S. Paschen (e), M. de Boissieu (f), Phys. Rev. Lett. 113, 025506 (2014).
(a) Institute of Light and Matter, UMR5306 Université Lyon 1-CNRS, Université de Lyon, Villeurbanne (France)
(b) Institute of Materials Science and Technology, Vienna University of Technology (Austria)
(c) Centre de Thermique de Lyon CETHIL UMR5008, UCBL, INSA de Lyon, CNRS (France)
(d) ESRF
(e) Institute of Solid State Physics, Vienna University of Technology (Austria)
(f) SIMAP, UJF, CNRS, INP Grenoble, St Martin d’Hères (France)
References
[1] M. Marek Koza, M.R. Johnson, R. Viennois, H. Mutka, L. Girard and D. Ravot, Nature Materials 7, 805 (2008).



